This course will take place in the context of the EECI International Graduate School on Control from the 8th of Aril to the 12th of April 2024 at Supmicrotech Besançon France.
Registration: https://eeciigsc.web-events.fr/earlyregistrationm03tom18
 |  |
 |  |
 |  |
Course abstract
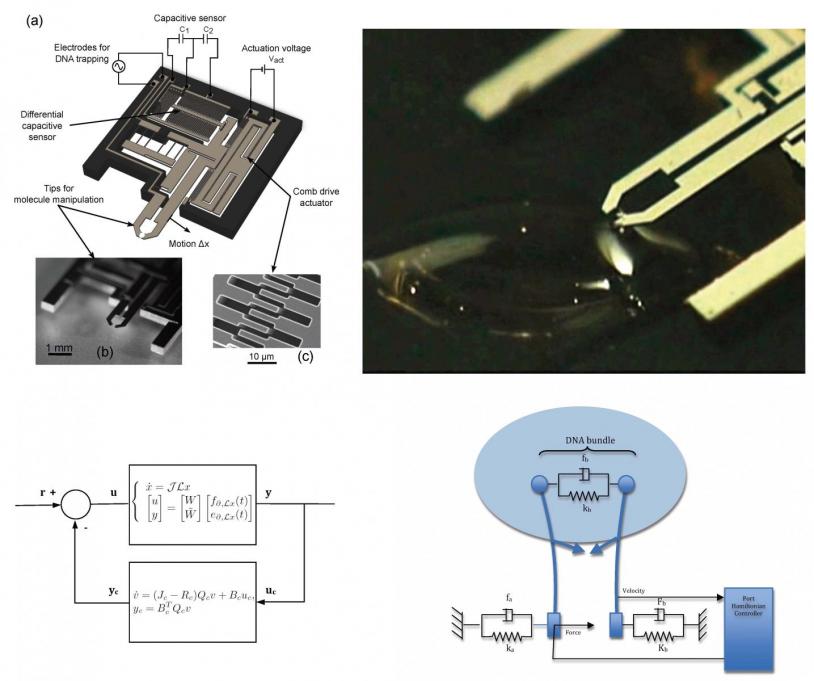
This course presents a system control-oriented approach to modeling, analysis, and control of distributed parameter systems (DPS), i.e., systems governed by partial differential equations (PDEs). This class of systems is more and more encountered in control engineering due to the increased use of complex, heterogeneous and smart materials in applications. (see Figure below).
Analysis and control of this class of systems is thus of high theoretical and practical interest, especially when considering the evolution of computing capacities that allows to deal with very high order systems. The formalism used in this course is the port-Hamiltonian framework. It formalizes the basic interconnection laws together with the power-conserving elements by a geometric (interconnection) structure, and defines the Hamiltonian function as the total energy stored in a system. The aim of this course is to show how this formalism can be advantageously used to study stability and derive simple and physically consistent control laws for the stabilization of linear infinite dimensional systems. The different parts of this course are also illustrated through physical examples such as electro-magnetic systems, transmission lines, beam equations, linearized shallow water equations, Korteweg-de Vries equations, reaction-transport problems including chemical processes, population dynamics, etc.
Organization
The organization of the cours is the followig:
- The first part of the course is devoted to modelling. More precisely, it focuses on the derivation of structured models accounting for power exchanges occurring within the system and with its environment.
- In the second part, existence of solutions, boundary control and stability of linear port-Hamiltonian systems are studied.
- The third part is concerned with control design.
The course ends with a tutorial aiming at applying the different concepts on a practical and realistic example in order to illustrate with simulations the interest of such an approach. The different parts of this course are also illustrated through physical examples such as transmission lines, beam equations, linearized shallow water equations, Korteweg-de Vries equations, reaction-transport problems including chemical processes, population dynamics, etc.